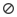Chủ đề này có 4 trả lời

[Zaraki]

Cho $n$ là một số nguyên dương, $p$ là một số nguyên tố thoả $p \equiv 7 \pmod{8}$. Hãy chứng minh rằng $$\sum_{k=1}^{p-1} \left \{ \frac{k^{2^n}}{p}+ \frac 12 \right \} = \frac{p-1}{2}.$$

 

Mình vừa nghĩ ra lời giải khác lời giải trong sách cho bài toán này.

Bài viết đã được chỉnh sửa nội dung bởi Zaraki: 03-01-2016 - 18:52

[hxthanh]

Cái ngoặc "{…}" của Toàn có ý nghĩa là gì vậy? $p$ là số nguyên tố?

[Zaraki]

Cái ngoặc là phần lẻ thầy ạ. $p$ là số nguyên tố thoả mãn $p \equiv 7 \pmod{8}$ ạ. Em quên mất điều kiện của $p$, để em thêm vào.

[Chris yang]

Cho $n$ là một số nguyên dương, $p$ là một số nguyên tố thoả $p \equiv 7 \pmod{8}$. Hãy chứng minh rằng $$\sum_{k=1}^{p-1} \left \{ \frac{k^{2^n}}{p}+ \frac 12 \right \} = \frac{p-1}{2}.$$

 

Mình vừa nghĩ ra lời giải khác lời giải trong sách cho bài toán này.

Bài toán tương đương với chứng minh 

$A=\sum_{k=1}^{p-1}\left \lfloor \frac{k^{2^n}}{p}+\frac{1}{2} \right \rfloor=\frac{1^{2^n}+2^{2^n}+...+(p-1)^{2^n}}{p}$

Áp dụng bổ đề quen thuộc là $\left \lfloor a \right \rfloor+\left \lfloor a+\frac{1}{2} \right \rfloor=\left \lfloor 2a \right \rfloor\Rightarrow A=\sum_{k=1}^{p-1}\left \lfloor \frac{2k^{2^n}}{p} \right \rfloor-\sum_{k=1}^{p-1}\left \lfloor \frac{k^{2^n}}{p} \right \rfloor$

Vì $p=8k+7$ nên $\left ( \frac{2}{p} \right )=1$, nên với mỗi $k_1\in (1,2,...,p-1)$ tồn tại duy nhất $k_2\in (1,2,...,p-1)$ mà $k_1^{2^n}\equiv 2k_2^{2^n}\pmod p$

Do đó, ta có thể viết $A=\sum \left ( \left \lfloor \frac{2k_i^{2^n}}{p} \right \rfloor-\left \lfloor \frac{k_j^{2^n}}{p} \right \rfloor \right )$ mà $p|2k_i^{2^n}-k_j^{2^n}$ ( có tất cả $p-1$ nhóm như thế)

Lại có $a,b\not\in\mathbb{Z},a-b\in\mathbb{Z}\Rightarrow \left \lfloor a \right \rfloor-\left \lfloor b \right \rfloor=a-b\Rightarrow A=\sum_{k=1}^{p-1}\frac{k^{2^n}}{p}$

Ta có đpcm

 

----------------------------------------------------------------------------------

P.s: Bài toán quen thuộc về tính tổng phần nguyên Không biết cách này có khác cách trong sách của bạn không?

[Zaraki]

Cảm ơn bạn, sau đây là lời giải của mình. Nó cực dài, và tính đúng sai còn chưa chắc chắn.
 
Lời giải. Đầu tiên, ta cần chứng minh cho $0 \le x \le 1$ then $$\left \{ x+ \frac 12 \right \} - \left \{ x \right \} = \begin{cases} \frac 12 \; \text{if} \; x \le \frac 12, \\ \frac{-1}{2} \; \text{if} \; x > \frac 12. \end{cases}$$
Điều này tương đương với that $$\left \{ \frac{k^{2^n}}{p}+ \frac 12 \right \} - \left \{ \frac{k^{2^n}}{p} \right \} = \begin{cases} \frac 12 \; \text{if} \; k^{2^n} \equiv a \pmod{p}, a \le \frac{p-1}{2}, \\ \frac{-1}{2} \; \text{if} \; k^{2^n} \equiv a \pmod{p}, p-1 \ge a \ge \frac{p+1}{2}. \end{cases} \qquad (1)$$
Gọi $x$ là số các số chính phương (mod $p$) mà $\le \tfrac{p-1}{2}$, $y$ là số các số chính phương (mod $p$) mà $\ge \tfrac{p+1}{2}$ thì $x+y= \tfrac{p-1}{2}$. Từ $(1)$ ta có $$\begin{aligned} \left \{  \frac{k^{2^n}}{p} + \frac 12 \right \} & = \left \{  \frac{k^{2^n}}{p} \right \} + (x-y), \\ & = \frac{2 \sum a}{p}+(x-y), \; \text{where} \; \left( \tfrac ap \right)=1, 1 \le a \le p-1, \\ & = \frac{2 \left( \sum a -yp \right)}{p}+ x+y, \\ & = \frac{2 \left( \sum a - yp \right)}{p}+ \frac{p-1}{2}. \end{aligned}$$
Bây giờ ta cần chứng minh $\sum a = py$. 
Kí hiệu $X,Y$ lần lượt là tổng các số chính phương mod $p$ mà $\le \tfrac{p-1}{2}, \ge \tfrac{p+1}{2}$;
Kí hiệu $\underset{\equiv m \pmod n, \; \le (p-1)/2 }{ \Large A}$ là tổng các số chính phương $\equiv m \pmod n$ và $\le (p-1)/2$. 
 
Ta sẽ đi chứng minh rằng $\underset{\equiv 1 \pmod 2}{ \Large A} = Y-X. \qquad (2)$
 

Spoiler

Để ý $\left( \frac 2p \right)=1$ since $p \equiv 7 \pmod{8}$. Ta có một số nhận xét sau:
 
1) $\underset{\equiv 0 \pmod{2^{i-1}}, \; \le t }{ \Large A} = \underset{\equiv 2^{i-1} \pmod{2^{i}}, \; \le t}{ \Large A} + \underset{\equiv 0 \pmod{2^i}, \; \le t }{ \Large A}.$
 
2) $2 \times \underset{\equiv 2^{i-1} \pmod{2^i}, \; \le t }{ \Large A}  = \underset{\equiv 2^i \pmod{2^{i+1}}, \; \le 2t }{ \Large A}.$
 
 
Quay lại bài toán: 

 

 

 

 

 

Điều cuối đúng dựa vào 2).

Để ý rằng cho $\tfrac{p+1}{2} \le a \le p-1$ và $\left( \frac{2a-p}{p} \right)= \left( \frac ap \right)$ thì $1 \le 2a-p \le p-1$ và $2 \nmid 2a-p$. Do đó 
$$2 \times Y - p \times y = \underset{\equiv 1 \pmod 2}{ \Large A} . \qquad (3)$$
Từ $(2)$ và $(3)$ ta suy ra $$py=Y+X= \sum a.$$
Vậy, bài toán được chứng minh. $\blacksquare$

Bài viết đã được chỉnh sửa nội dung bởi Zaraki: 22-02-2016 - 03:23